Imaginary Roots Characteristic Equation. Y 10y 29 0 y 0 1 y 0 3. This system is stable sys.
This system is stable sys. Then the general solution to the differential equation is given by y e lt c 1 cos mt c 2 sin mt example. Galois approach via imaginary roots and dedekind s approach via residue class rings were shown to be essentially equivalent by kronecker.
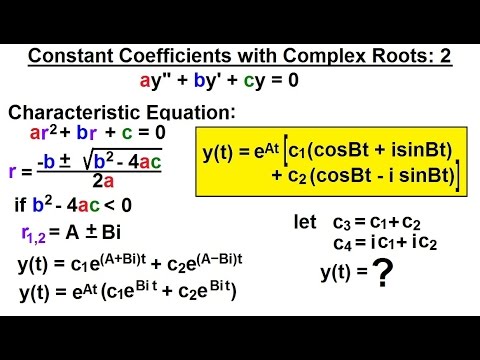
In this section we discuss the solution to homogeneous linear second order differential equations ay by c 0 in which the roots of the characteristic polynomial ar 2 br c 0 are complex roots.
The roots of the reverse characteristic equation are not real. 1 if all the roots are lying on the left hand side of the s plane then this system is stable system for example 1 s 1. Galois approach via imaginary roots and dedekind s approach via residue class rings were shown to be essentially equivalent by kronecker. Hi there are three types stability according to the position of roots of characteristics equation.